Isometric sheets
If you crumple a sheet of paper, a number of ridges form. They come together at points resembling miniature cones (see Fig. 1); remarkably, these point defects tell the paper how to fold. This can be illustrated very beautifully: just depress a flat planar disc of paper with a pencil into your coffee cup and observe how the disc deforms.
 |
 |
| Fig. 1: Crumpled paper |
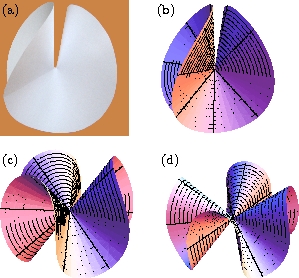
Fig. 2: The e-cone |
Conical defects in crumpled paper are quite different from the familiar icecream cone one obtains by removing a wedge and glueing the sides of the remaining disc together. Surprisingly, if an extra wedge is added to the disc, an infinite number of shapes arises without any need to apply a force.
Possible shapes of such an excess cone are illustrated in Fig. 2.
In nature certain seawater algae, for example, spontaneously adopt these shapes during their development.
With the help of differential geometry a simplified model of the e-cone is presented. The results compare very well with simple experiments on sheets of paper. It turns out that the configuration with the twofold symmetry is the ground state.
Relevant publications
- Isometric bending requires local constraints on free edges
Jemal Guven, Martin Michael Müller, Pablo Vázquez-MontejoWhile the shape equations describing the equilibrium of an unstretchable thin sheet that is free
to bend are known, the boundary conditions that supplement these equations on free edges have remained elusive.
Intuitively, unstretchability is captured by a constraint on the metric within the bulk. Naïvely one would then
guess that this constraint is enough to ensure that the deformations determining the boundary conditions on these
edges respect the isometry constraint. If matters were this simple, unfortunately, it would imply unbalanced torques
(as well as forces) along the edge unless manifestly unphysical constraints are met by the boundary geometry. In this
article, we identify the source of the problem: not only the local arc-length but also the geodesic curvature need to
be constrained explicitly on all free edges. We derive the boundary conditions which follow. In contrast to conventional
wisdom, there is no need to introduce boundary layers. This framework is applied to isolated conical defects, both
with deficit as well, but more briefly, as surplus angles. Using these boundary conditions, we show that the lateral
tension within a circular cone of fixed radius is equal but opposite to the radial compression, and independent of
the deficit angle itself. We proceed to examine the effect of an oblique outer edge on this cone perturbatively
demonstrating that both the correction to the geometry as well as the stress distribution in the cone kicks in at
second order in the eccentricity of the edge.
Math. Mech. Solids, 24: 4051, 2019. See also arXiv:1904.05855.
- Dipoles in thin sheets
Jemal Guven, J. A. Hanna, Osman Kahraman, Martin Michael MüllerA flat elastic sheet may contain pointlike conical singularities that carry a metrical "charge" of Gaussian curvature. Adding such elementary defects to a sheet allows one to make many shapes, in a manner broadly
analogous to the familiar multipole construction in electrostatics. However, here the underlying field theory is non-linear,
and superposition of intrinsic defects is non-trivial as it must respect the immersion of the resulting surface in three
dimensions. We consider a "charge-neutral" dipole composed of two conical singularities of opposite sign.
Unlike the relatively simple electrostatic case, here there are two distinct stable minima and an infinity of unstable equilibria.
We determine the shapes of the minima and evaluate their energies in the thin-sheet regime where bending dominates
over stretching. Our predictions are in surprisingly good agreement with experiments on paper sheets.
Eur. Phys. J. E, 36: 106, 2013. See also arXiv:1212.3262.
- Conical instabilities on paper
Jemal Guven, Martin Michael Müller, Pablo Vázquez-MontejoThe stability of the fundamental defects of an unstretchable flat sheet is examined.
This involves expanding the bending energy to second order in deformations about the
defect. The modes of deformation occur as eigenstates of a fourth-order linear differential
operator. Unstretchability places a global linear constraint on these modes. Conical
defects with a surplus angle exhibit an infinite number of states. If this angle is below a
critical value, these states possess an n-fold symmetry labeled by an integer, n ≥ 2. A
nonlinear stability analysis shows that the 2-fold ground state is stable, whereas excited
states possess 2(n - 2) unstable modes which come in even and odd pairs.
J. Phys. A: Math. Theor., 45(1): 015203, 2012. See also arXiv:1107.5008.
- Self-Contact and Instabilities in the Anisotropic Growth of Elastic Membranes
Norbert Stoop, Falk K. Wittel, Martine Ben Amar, Martin Michael Müller, Hans J. HerrmannWe investigate the morphology of thin discs and rings growing in circumferential direction. Recent analytical results suggest that this growth produces symmetric excess cones (e-cones). We study the stability of such solutions considering self-contact and bending stress. We show that, contrary to what was assumed in previous analytical solutions, beyond a critical growth factor, no symmetric e-cone solution is energetically minimal any more. Instead, we obtain skewed e-cone solutions having lower energy, characterized by a skewness angle and repetitive spiral winding with increasing growth. These results are generalized to discs with varying thickness and rings with holes of different radii.
Phys. Rev. Lett., 105(6): 068101, 2010. See also arXiv:1007.1871.
- Conical Defects in Growing Sheets
Martin Michael Müller, Martine Ben Amar, Jemal GuvenA growing or shrinking disc will adopt a conical shape, its intrinsic geometry characterized by a surplus angle φe at the apex. If growth is slow, the cone will find its equilibrium. Whereas this is trivial if φe≤0, the disc can fold into one of a discrete infinite number of states if φe is positive. We construct these states in the regime where bending dominates, determine their energies and how stress is distributed in them. For each state a critical value of φe is identified beyond which the cone touches itself. Before this occurs, all states are stable; the ground state has twofold symmetry.
Phys. Rev. Lett., 101(15): 156104, 2008. See also arXiv:0807.1814.
- How paper folds: bending with local constraints
Jemal Guven, Martin Michael MüllerA variational framework is introduced to describe how a surface bends when it is subject to local constraints on its geometry. This framework is applied to describe the patterns of a folded sheet of paper. The unstretchability of paper implies a constraint on the surface metric; bending is penalized by an energy quadratic in mean curvature. The local Lagrange multipliers enforcing the constraint are identified with a conserved tangential stress that couples to the extrinsic curvature of the sheet. The framework is illustrated by examining the deformation of a flat sheet into a generalized cone.
J. Phys. A: Math. Theor., 41(5): 055203, 2008. See also arXiv:0712.0978.
|





